INITIAL ABSTRACTION REVISITED Victor M. Ponce and Luis Magallon
|
|
The concept of initial abstraction in the NRCS runoff curve number method is revisited, in light of the demonstrably different results obtained by current models (SWMM and HEC-HMS). The runoff curve number method is lumped in time, but it has been used by way of practice as a temporally distributed model, beyond the scope of its original development. This has led to various ways of accounting for the initial abstraction. There is an urgent need to revise the concept and to develop a new standard, so that the various models will lead to the same or similar results. |
The concept of initial abstraction has evolved since its introduction in the 1950s as part of the NRCS runoff
curve number method (Ponce, 2014).
Several interpretations are in current use, which has defied standarization.
Hydrologic models such as SWMM and HEC-HMS apply the concept
in different ways, resulting in different answers. Thus, it has become
necesary to review and clarify the concept of initial abstraction in the runoff curve number method, in the hope that in the future,
when the method is used in conjunction with SWMM and HEC-HMS,
the calculated hydrograph will be essentially the same.
The concept of initial abstraction originated with the NRCS runoff curve number (RCN) method, developed
by the USDA Natural Resources Conservation Service (formerly Soil Conservation Service, or SCS) in the 1950s, and documented in
National Engineering Handbook: Part 630 - Hydrology.
For a given storm depth P and runoff curve number CN,
the initial abstraction Ia is the initial fraction of
the storm depth after which runoff begins.
Victor Mockus, the lead developer of the RCN method, believed that for any one case, the amount of
initial abstraction was, for all practical purposes, intractable.
To circumvent the problem, Mockus proposed to plot
As Mockus told Ponce in the now famous
interview (Ponce, 1996),
he was overruled by his superiors at SCS, who believed that
an initial abstraction component had to be part of the nascent RCN method.
For completeness, the original runoff curve number equation developed by Mockus is:
P - Q Q in which
S = potential storage, with all variables taken in depth units.
The initial abstraction Ia
was subtracted from the storm depth P to yield:
P - Ia - Q Q
To keep the method simple,
Mockus expressed the potential retention S in terms of a more manageable
1000
in which CN is a dimensionless number limited in the range 1 ≤ CN ≤ 100, and all other terms in
For the method to remain practical,
featuring only one parameter (CN),
the initial abstraction had to be
related to the storage S.
After much discussion, the following linear relation was adopted:
Ia = λ S
in which λ = initial abstraction parameter.
At the method's inception, the value of
At the present time (2020), no decision has been made by NRCS on whether to keep or to change the
value of λ. Since the current set of CN's were developed in conjunction with
In the time elapsed since its original development,
the NRCS runoff curve number method has become a
Mockus pointed out that the method was not intended to be a predictor of the rate of infiltration,
but rather, of the total volume of infiltration for a given storm event (Ponce, 1996).
Given P (in inches) and an estimate of CN, the runoff volume Q (in inches) is
(Ponce, 2014):
[ CN ( P + 2 ) - 200 ] 2
As originally proposed, the NRCS RCN method is effectively lumped
in time, providing an estimate of storm runoff Q (in), given storm rainfall P (in)
and an appropriate CN value, independently of the storm duration,
which is not explicitly accounted for.
Mockus stated that the supporting data used in the method's development
was daily rainfall, because this type of data was the only one available in large quantities
(Ponce, 1996).
In practice, this means that the RCN method ought to work best when the
storm duration is close to one (1) day,
although in many applications this has not been necessarily
the case (NRCS, 1986).
The correct definition of initial abstraction in the NRCS RCN method follows from the
method's original development:
"For a given storm depth P and runoff curve number CN,
the initial abstraction Ia is the fraction of the storm depth after
which runoff begins."
Note that in the RCN method, the initial abstraction serves the avowed purpose
of reducing the runoff Q below
the value which would apply had the initial abstraction been zero.
The emphasis is on the effect of initial abstraction in reducing total runoff Q (ordinate),
and not on applying the initial abstraction to rainfall (abscissa).
According to the RCN method,
runoff Q is a function of storm depth P
and curve number CN, regardless of the storm duration.
Therefore, for a given curve number CN,
the storage S and
initial abstraction Ia are constants.
Like P, S, and Q, the initial abstraction Ia is a volume, interpreted as a fraction
of storm depth evenly distributed for the given storm duration and watershed under consideration.
It is argued here that if CN and S do not account for either the rate of infiltration or the storm duration,
neither should the initial abstraction Ia. On this premise,
it appears unwarranted to place the initial abstraction
at the beginning of the storm.
We observe that the RCN method is lumped in time, originally developed for
storm durations of 24 hr, and subsequently extended by way of practice
to storms of lesser duration.
Given tr = storm duration, tc =
time of concentration, and Pe = effective precipitation,
distributing the total abstraction (P - Q) (i.e., the total losses) uniformly in time
produces a constant effective rainfall intensity (Ie
= Pe /tr). For
tr ≥ tc,
this procedure leads to runoff concentration, with peak flow Qp1
(Ponce, 2014):
Qp1 = Ie A
Conversely, if the initial abstraction is accounted for in the beginning of the storm,
the total abstraction (P - Q) is not distributed uniformly in time.
Thus, in order to conserve mass throughout the storm duration, runoff concentration must be attained
at a peak flow Qp2, where:
Qp2 > Qp1
with significant differences between the two approaches.
Figure 1 shows a typical example of the difference in peak flows (Magallon and Ponce, 2015).
The question remains as to
which of the approaches to rainfall abstraction using
the runoff curve number method is more realistic or more appropriate.
This results is significant differences in hydrograph properties
(Magallon and Ponce, 2015).
Fig. 1 Comparison of hydrographs for three overland flow models, using
The concept of initial abstraction in the NRCS runoff curve number method is revisited,
in light of the demonstrably different results
obtained by hydrologic models in current use (SWMM and
REFERENCES
Hawkins, R. H., R. Jiang, D. E. Woodward, A. T. Hjelmfelt, and J. E. VanMullen, 2002.
Runoff curve
number method: Examination of the initial abstraction ratio. Proceedings of the Second Federal Interagency
Hydrologic Modeling Conference, Las Vegas, Nevada.
Magallon, L., and V. M. Ponce. 2015. Comparison between overland flow models.
Online publication.
Ponce, V. M. 1996. Notes of my conversation with Vic Mockus. Online feature.
Ponce, V. M. 2014. Engineering Hydrology, Principles and Practices. Online edition.
Ponce, V. M., and R. H. Hawkins. 1996.
Runoff curve number: Has it reached maturity?
ASCE Journal of Hydrologic Engineering, Vol. 1, No. 1, January, 11-19.
USDA Natural Resources Conservation Service. 1986.
Urban Hydrology for Small Watersheds.
USDA Natural Resources Conservation Service. 2015.
National Engineering Handbook: Part 630 - Hydrology.
_______ = ______
S P
(1)
___________ = _________
S P - Ia
(2)
S = _________ - 10
CN
(3) (4)
Q = ___________________________
CN [ CN ( P - 8 ) + 800 ]
(5)
(6) (7)
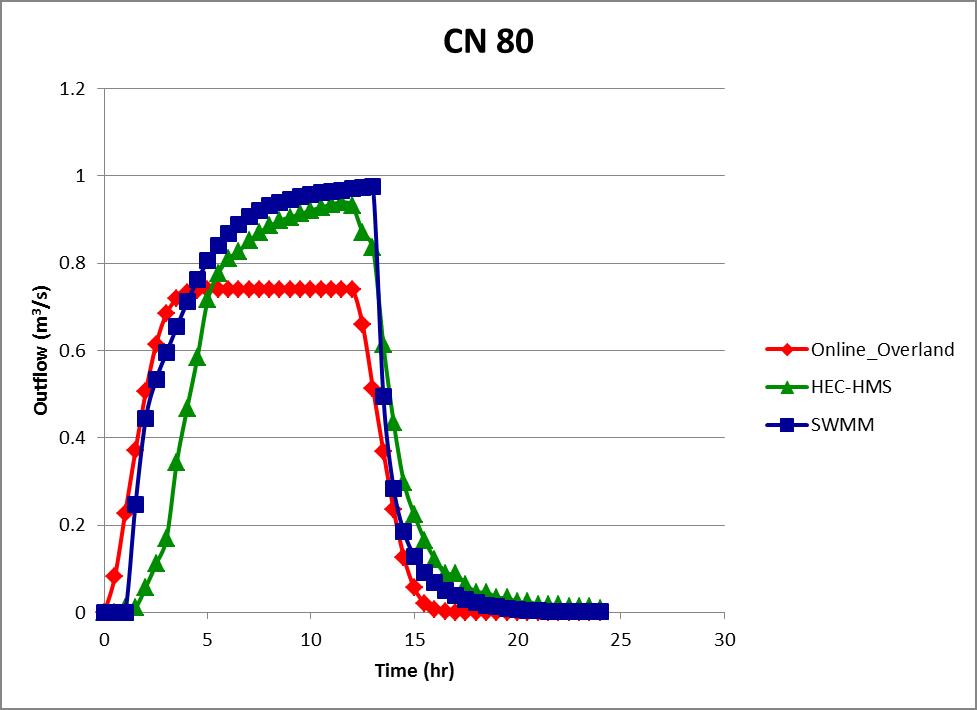
the NRCS runoff curve number
as the abstraction method.