1.  INTRODUCTION

This paper contrasts kinematic and dynamic waves in open-channel flow. The objective is to understand these concepts thoroughly, in order to facilitate their wider use in engineering practice. Kinematic and dynamic waves lie each at either extreme of the wave scale, i.e., the dimensionless wavenumber spectrum. Kinematic waves are on the left side (small value), and dynamic waves are on the right side (large value). Taken on their own, these two concepts are mutually exclusive; either a wave is kinematic or it is dynamic. Toward the middle of the scale, a wave that is neither kinematic nor dynamic may be construed as being a mixed kinematic-dynamic wave, for lack of a better term. The varying group celerity makes these waves diffusive, ranging from mildly diffusive to extremely diffusive.

2.  WAVE SCALE

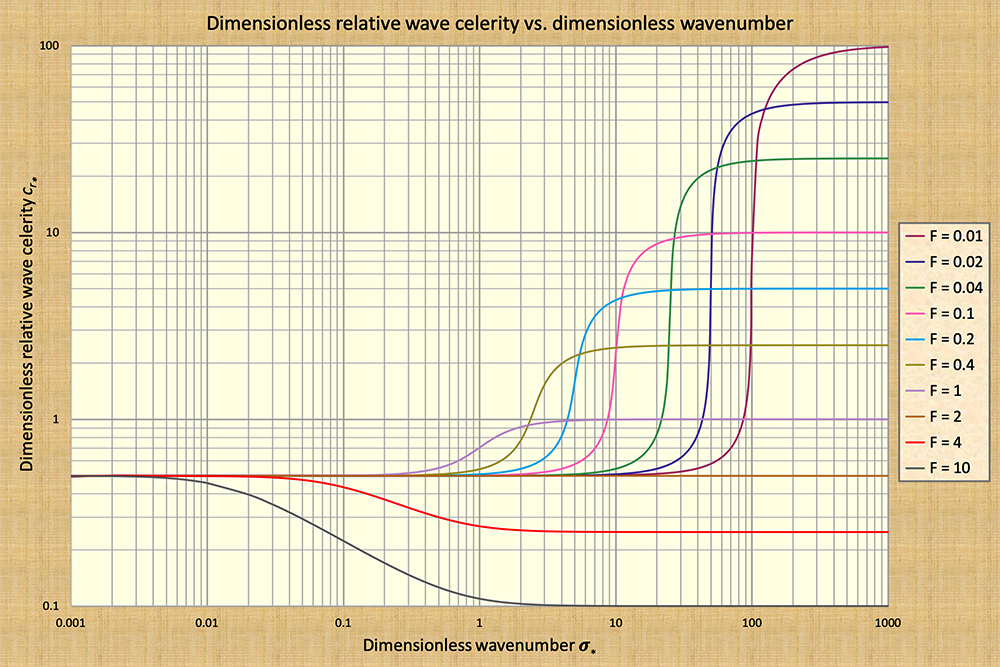
The "scale" of the wave is what determines whether a wave is either kinematic or dynamic. In this context, "scale" refers not to the absolute value of wavenumber, otherwise defined as σ = 2π /L, but rather to its relative value, or dimensionless wavenumber, defined as σ_* = 2π (L_o /L). The quantity L_o is the reference channel length, i.e., the horizontal distance, along the channel, wherein the steady equilibrium flow drops a head equal to its depth (Ponce and Simons, 1977).

The effect of the dimensionless wavenumber is to substantially reduce the number of orders of magnitude required for the analysis. In effect, Figure 1 shows the variation of dimensionless relative wave celerities c_r* plotted across only six (6) orders of magnitude of dimensionless wavenumbers σ_*  (0.001 to 1000).

Fig. 1   Dimensionless relative wave celerity cr* vs dimensionless wavenumber σ*.

---

3.  KINEMATIC WAVES

Box A.  To facilitate the understanding of this section, we define the following terms: Equilibrium flow depth do Equilibrium flow velocity uo Stream/channel bottom slope So Wavelength (of the perturbation) L Wavenumber σ = 2π / L Reference channel length Lo = do /So Dimensionless wavenumber σ* = 2π (Lo /L) Wave celerity (speed of the perturbation) c Relative wave celerity (wave celerity relative to the flow) cr = c - uo Dimensionless relative wave celerity cr* = cr /uo

Figure 1 shows the plot of relative dimensionless wave celerities across the dimensionless wavenumber spectrum, from very small, corresponding to kinematic waves (σ_* = 0.001), to very large, corresponding to dynamic waves (σ_* = 1000) (Ponce and Simons, 1977).

Note that throughout the dimensionless wavenumber spectrum σ_*, the dimensionless relative wave celerity c_r* is a constant and equal to c_r* = 0.5 only for Froude number F = 2. We note specifically that this flow condition corresponds to Chezy friction in a hydraulically wide channel (se Box B below) (Ponce and Simons, 1977). This is the physical condition for which all wave scales travel with the same celerity, representing the onset of flow stability/instability: stability for F < 2, and instability F > 2. Note that the flow condition for which F = 2 is referred to as neutrally stable flow. Moreover, it can be shown that the condition Froude number F = 2 is tantamount to Vedernikov number V = 1, thus, reinforcing the findings of the wave propagation analysis (Ponce, 1991).

Fig. 2   Dimensionless relative wave celerity cr* vs dimensionless wavenumber σ*.

Further examination of Fig. 1 reveals that the kinematic waves are positioned to the left of the figure, in a fashion asymptotic to the constant value c_r* = 0.5 in the extreme left of the figure, which corresponds to the dimensionless relative kinematic wave celerity for Chezy friction in a hydraulically wide channel (Ponce, 2014).

The concept of kinematic wave celerity, which is akin to that of flood wave celerity, is due to Seddon (1900), who first derived the formula bearing his name. Related expressions are contained in the following box.

Box B.  Expressions for kinematic wave celerity, Seddon celerity, or flood wave celerity. Discharge Q Flow area A Mean flow velocity: uo = Q / A Stage y Channel top width T Differential of flow area: dA = T dy Equation of the discharge-flow area rating: Q = α Aβ Slope of the discharge-flow area rating: dQ / dA = α β A β - 1 = β Q / A = β uo Seddon celerity, or flood wave celerity: c = dQ / dA = (1/T ) (dQ / dy) = β uo Value of β applicable for Chezy friction in hydraulically wide channels: β = 1.5 Relative flood wave celerity (for hydraulically wide Chezy friction): cr = 1.5 uo - uo = 0.5 uo Dimensionless relative kinematic wave celerity (for hydraulically wide Chezy): cr* = 0.5

We wish to reiterate that kinematic waves do exist, admittedly only as a convenient approximation, typically on the left side of the dimensionless wavenumber spectrum. They correspond to a large class of flood waves, particularly those that are subject to very little (or otherwise, negligible) attenuation. They also may show up in overland flow modeling, wherein the prevailing bottom slopes are large enough to trigger a kinematic flow condition and the resulting kinematic waves (Woolhiser and Liggett, 1967). The early work of Seddon (1900), followed by that of Lighthill and Whitham (1955), have been important milestones in advancing kinematic wave applications in hydraulic and hydrologic engineering.

---

4.  DYNAMIC WAVES

Dynamic waves lie to the right of the dimensionless wavenumber spectrum, and the dimensionless relative wave celerities are constant across the dimensionless wavenumbers, and a function of the Froude number of the equilibium flow, with smaller celerities corresponding to larger Froude numbers, and vice versa; for instance, c_r* = 1 is associated with F = 1; and c_r* = 100 is associated with F = 0.01. Clearly, the dynamic wave celerity is indeed a function of the equilibrium flow Froude number, a situation which was not the case for the kinematic wave.

Box C.  To calculate the values of dynamic wave celerities, we define the following terms: Equilibrium flow depth do Equilibrium flow velocity uo Gravitational acceleration g Dynamic wave celerity, or Lagrange celerity (two components) cd = uo ± (g do)1/2 Relative dynamic wave celerity (relative to the flow) crd = ± (g do)1/2 Dimensionless relative dynamic wave celerity cdrd = ± (g do)1/2 / uo Froude number of the equilibrium flow Fo = uo / (g do)1/2 Dimensionless relative dynamic wave celerity cdrd = 1 / Fo

Figure 1 shows the values of dimensionless relative dynamic wave celerities c_drd lying to the right of the figure. For instance, using the last definition of c_drd (labeled 8 in Box C above), it follows that: (a) for F_o = 0.01, c_drd = 100; (b) for F_o = 0.02, c_drd = 50; and (c) for F_o = 0.04, c_drd = 25; and so on.

We have shown that Figure 1 correctly depicts the values of dimensionless relative dynamic, or Lagrange, celerities. Thus, we show that Figure 1 encompasses both kinematic and dynamic waves in the same figure. Reiterating, dynamic waves lie to the right of the dimensionless wavenumber σ_*, wherein the dimensionless relative dynamic wave celerities are a function of the Froude number of the equilibrium flow.

According to Ponce and Simons (1977), the attenuation of a dynamic wave is zero, i.e., dynamic waves are not subject to attenuation (i.e., wave dissipation), at least in a one-dimensional analysis. This conclusion follows directly from Fig. 1, because in the applicable dynamic range, toward the extreme right of the figure, the wave celerity is shown to be constant and, thus, independent of scale. This conclusion confirms that a dynamic wave is not subject to attenuation. Thus, a dynamic wave is a comparatively small surface wave, featuring a correspondingly small dimensionless wavenumber, traveling at a dimensionless relative celerity which is the reciprocal of the Froude number of the equilibrium flow, and it is not subject to attenuation.

Dynamic waves do exist, admittedly only as a convenient approximation, typically on the right side of the dimensionless wavenumber spectrum. They correspond to a class of relatively short surface waves, particularly those that are subject to very little or negligible attenuation.

Fig. 1   Dimensionless relative wave celerity cr* vs dimensionless wavenumber σ*.

Fig. 2   Logarithmic decrement -δ vs dimensionless wavenumber σ*  for F < 2.

Fig. 3   Logarithmic increment +δ vs dimensionless wavenumber σ*  for F > 2.

Therefore, mixed kinematic-dynamic waves are subject to varying attenuation, from mild to very strong, with the amount of attenuation varying with the value of dimensionless wavenumber, relative to the location of the point of inflection in the celerity-wave number curve. In certain cases, the mixed kinematic-dynamic wave may be so strongly dissipative as to defy calculation altogether. This predicament was admirably described by Lighthill and Whitham (1955) in their seminal treatise on kinematic waves.

In closing, we wish to point out that our "mixed kinematic-dynamic waves" have been, for the past nearly 50 years, simply referred to as "dynamic waves", thereby contributing to the semantic confusion (Fread, 1985).

6.  DIFFUSION WAVES

Having established conclusively that neither kinematic nor dynamic waves attenuate, and conversely, that mixed kinematic-dynamic waves could be subject to very strong attenuation, we feature here another type of intermediate wave, which, as far as the value of dimensionless wavenumber, lies in between kinematic and mixed kinematic-dynamic waves. This wave is properly a kinematic wave with diffusion, to follow Lighthill and Whitham (1955), or, more concisely, a diffusion wave, to follow Ponce and Simons (1977). It is defined by including, in the wave definition, the pressure-gradient term. The latter acts to produce the diffusion, which is patently absent from the kinematic wave proper, as shown in the following table.

Diffusion waves turn out to be more common than either kinematic or mixed kinematic-dynamic waves and, therefore, this helps explain their growing popularity in practical applications. Kinematic waves do not attenuate, and mixed kinematic-dynamic waves may actually attenuate too much. Diffusion waves find their demonstrably best applicability in the routing of flood waves, which typically are subjected to some, but not too much, wave attenuation.

7.  SUMMARY

A review of several relevant types of shallow-water waves in open-channel flow are discussed and compared with regard to their celerity and attenuation properties. These waves are: (1) kinematic waves, (2) dynamic waves, (3) mixed kinematic-dynamic waves, and (4) diffusion waves.

Kinematic waves travel with a constant celerity and are non-diffusive. The constant celerity of kinematic waves has been referred to in the flood routing literature as the 'Seddon celerity'.

Dynamic waves travel with a constant celerity and are non-diffusive. The constant celerity of dynamic waves is referred to as the 'Lagrange celerity', applicable to "short" waves in open-channel flow.

Mixed kinematic-dynamic waves travel with a celerity which varies with the dimensionless wavenumber, and this property gives them the capability to diffuse, i.e., to attenuate or dissipate. In certain cases, these mixed kinematic-dynamic waves may be so strongly dissipative as to defy calculation altogether.

Diffusion waves lie in between kinematic and mixed kinematic-dynamic waves, in terms of relative scale. These waves travel approximately with the Seddon celerity and are shown to be mildly diffusive; therefore, they remain admirably suited to the modeling of flood waves.

REFERENCES

Fread, D. L. 1985. "Channel Routing," in Hydrological Forecasting, M. G. Anderson and T. P. Burt, eds. New York: John Wiley.

Ponce, V. M. and D. B. Simons. 1977. Shallow wave propagation in open channel flow. Journal of Hydraulic Engineering, ASCE, 103(12), 1461-1476.

Ponce, V. M. 1991. New perspective on the Vedernikov number. Water Resources Research, Vol. 27, No. 7, 1777-1779, July.

Ponce, V. M. 2014. Fundamentals of Open-channel Hydraulics. Online text.

Seddon, J. A. 1900. River hydraulics. Transactions, ASCE, Vol. XLIII, 179-243, June.

Woolhiser, D. A. and J. A. Liggett. 1967. Unsteady one-dimensional flow over a plane: The rising hydrograph. Water Resources Research, Vol. 3, 753-771.